Berechnen Sie die Größe des spitzen Winkels, den die Seitenfläche \(ABF\) und die Grundfläche \(ABCD\) einschließen.
(3 BE)
Lösung zu Teilaufgabe c
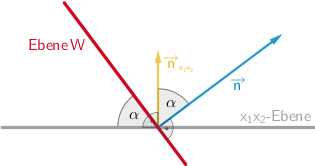
Die Größe des spitzen Winkels, den die Seitenfläche \(ABF\) und die Grundfläche \(ABCD\) einschließen, entspricht dem Schnittwinkel \(\alpha\) der Ebene \(W\) und der \(x_{1}x_{2}\)-Ebene. Dieser ist gleich der Größe des spitzen Winkels, den die Normalenvektoren der Ebenen einschließen (Skizze nicht verlangt).
Normalenvektor der Ebene \(W\): \(\textcolor{#0087c1}{\overrightarrow{n} = \begin{pmatrix} 0 \\ 4 \\ 3 \end{pmatrix}}\) (vgl. Teilaufgabe b)
Beispielsweise ist \(\textcolor{#e9b509}{\overrightarrow{n}_{\small{x_{1}x_{2}}} = \begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix}}\) ein Normalenvektor der \(x_{1}x_{2}\)-Ebene.
Schnittwinkel \(\boldsymbol{\alpha}\) zweier Ebenen
\[E_1\colon \enspace \overrightarrow{n}_1 \circ \left( \overrightarrow{X} - \overrightarrow{A} \right) = 0\]
\[E_2\colon \enspace \overrightarrow{n}_2 \circ \left( \overrightarrow{X} - \overrightarrow{B} \right) = 0\]
\[\cos \alpha = \frac{\vert \overrightarrow{n}_1 \circ \overrightarrow{n}_2 \vert}{\vert \overrightarrow{n}_1 \vert \cdot \vert \overrightarrow{n}_2 \vert} \enspace \Rightarrow \enspace \alpha = \cos^{-1}(\dots)\]
\[(0^{\circ} \leq \alpha \leq 90^{\circ})\]

\[\begin{align*} \cos \alpha &= \frac{\vert \textcolor{#0087c1}{\overrightarrow{n}} \circ \textcolor{#e9b509}{\overrightarrow{n}_{\small{x_{1}x_{2}}}} \vert}{\vert \textcolor{#0087c1}{\overrightarrow{n}} \vert \cdot \vert \textcolor{#e9b509}{\overrightarrow{n}_{\small{x_{1}x_{2}}}} \vert} = \frac{\left|\textcolor{#0087c1}{\begin{pmatrix} 0 \\ 4 \\ 3 \end{pmatrix}} \circ \textcolor{#e9b509}{\begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix}}\right|}{\left| \textcolor{#0087c1}{\begin{pmatrix} 0 \\ 4 \\ 3 \end{pmatrix}} \right| \cdot \left| \textcolor{#e9b509}{\begin{pmatrix} 0 \\ 0 \\ 1 \end{pmatrix}} \right|} \\[0.8em] &= \frac{\vert \textcolor{#0087c1}{0} \cdot \textcolor{#e9b509}{0} + \textcolor{#0087c1}{4} \cdot \textcolor{#e9b509}{0} + \textcolor{#0087c1}{3} \cdot \textcolor{#e9b509}{1}\vert}{\sqrt{\textcolor{#0087c1}{0}^2 + \textcolor{#0087c1}{4}^2 + \textcolor{#0087c1}{3}^2} \cdot \sqrt{\textcolor{#e9b509}{0}^2 + \textcolor{#e9b509}{0}^2 + \textcolor{#e9b509}{1}^2}} \\[0.8em] &= \frac{3}{\sqrt{25} \cdot \sqrt{1}} = \frac{3}{5} & &| \; \text{TR}\colon\, \cos^{-1}(\dots) \\[2.4em] \alpha &= \cos^{-1}\left( \frac{3}{5} \right) \approx 53{,}1^{\circ} \end{align*}\]


