Die Abbildung zeigt einen Würfel der Kantenlänge 6. Die Koordinaten der Eckpunkte \(A\,(0|0|0)\), \(D\,(0|6|0)\) und \(G\,(6|6|6)\) sind gegeben.
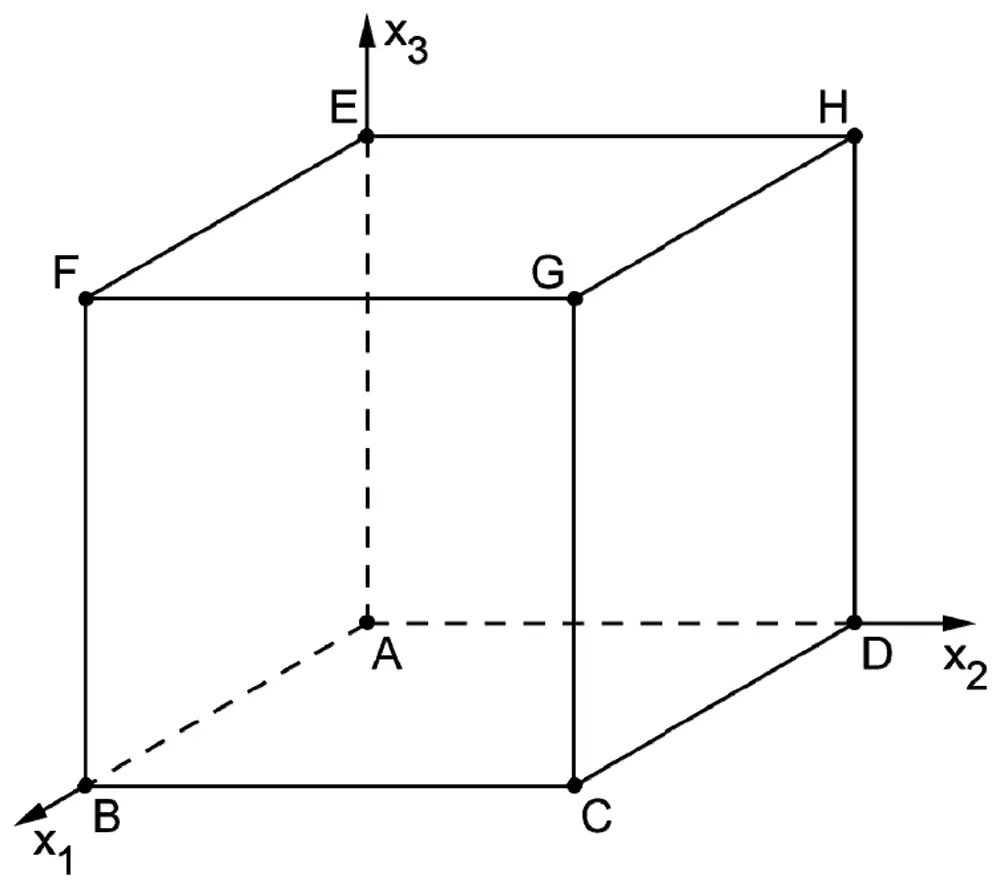
Die Punkte \(B\), \(E\) und \(G\) liegen in einer Ebene \(L\). Bestimmen Sie eine Gleichung von \(L\) in Normalenform. Zeichnen Sie die Figur, in der die Ebene \(L\) den Würfel schneidet, in die Abbildung ein.
(mögliches Ergebnis: \(L\,\colon\; x_1 - x_2 + x_3 = 6\))
(5 BE)
Lösung zu Teilaufgabe a
\[A\,(0|0|0), \enspace D\,(0|6|0), \enspace G\,(6|6|6)\]
Gleichung der Ebene \(L\) in Koordinatenform
Ebenengleichung in Normalenform (vgl. Merkhilfe)
Jede Ebene lässt sich durch eine Gleichung in Normalenform beschreiben. Ist \(A\) ein beliebiger Aufpunkt der Ebene \(E\) und \(\overrightarrow{n}_{E}\) ein Normalenvektor von \(E\), so erfüllt jeder Punkt \(X\) der Ebene \(E\) folgende Gleichungen:
Normalenform in Vektordarstellung
\[E \colon \overrightarrow{n}_{E} \circ (\overrightarrow{X} - \overrightarrow{A}) = 0\]
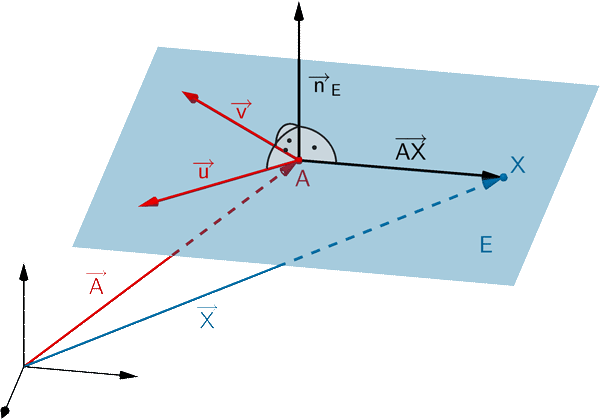
Normalenform in Koordinatendarstellung
\[E \colon n_{1}x_{1} + n_{2}x_{2} + n_{3}x_{3} + n_{0} = 0\]
mit \(n_{0} = -(\overrightarrow{n}_{E} \circ \overrightarrow{A}) = - \: n_{1}a_{1} - n_{2}a_{2} - n_{3}a_{3}\)
\(n_{1}\), \(n_{2}\) und \(n_{3}\): Koordinaten eines Normalenvektors \(\overrightarrow{n}_{E}\)
Richtungsvektoren der Ebene \(L\) bestimmen:
Der Abbildung können die Koordinaten der Punkte \(B\) und \(E\) entnommen werden.
\[B\,(6|0|0)\,\enspace E\,(0|0|6)\]
\[\overrightarrow{GE} = \overrightarrow{E} - \overrightarrow{G} = \begin {pmatrix} 0 \\ 0 \\ 6 \end {pmatrix} - \begin {pmatrix} 6 \\ 6 \\ 6 \end {pmatrix} = \begin {pmatrix} -6 \\ -6 \\ 0 \end {pmatrix}\]
\[\overrightarrow{GB} = \overrightarrow{B} - \overrightarrow{G} = \begin {pmatrix} 6 \\ 0 \\ 0 \end {pmatrix} - \begin {pmatrix} 6 \\ 6 \\ 6 \end {pmatrix} = \begin {pmatrix} 0 \\ -6 \\ -6 \end {pmatrix}\]
Normalenvektor der Ebene \(L\) bestimmen:
Vektorprodukt (Kreuzprodukt)
Das Vektorprodukt \(\overrightarrow{a} \times \overrightarrow{b}\) zweier Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) erzeugt einen neuen Vektor \(\overrightarrow{c} = \overrightarrow{a} \times \overrightarrow{b}\) mit den Eigenschaften:
\(\overrightarrow{c}\) ist sowohl zu \(\overrightarrow{a}\) als auch zu \(\overrightarrow{b}\) senkrecht.
\[\overrightarrow{c} = \overrightarrow{a} \times \overrightarrow{b} \quad \Longrightarrow \quad \overrightarrow{c} \perp \overrightarrow{a}, \enspace \overrightarrow{c} \perp \overrightarrow{b}\]
Der Betrag des Vektorprodukts zweier Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) ist gleich dem Produkt aus den Beträgen der Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) und dem Sinus des von ihnen eingeschlossenen Winkels \(\varphi\).
\[\vert \overrightarrow{a} \times \overrightarrow{b} \vert = \vert \overrightarrow{a} \vert \cdot \vert \overrightarrow{b} \vert \cdot \sin{\varphi} \quad (0^{\circ} \leq \varphi \leq 180^{\circ})\]
Die Vektoren \(\overrightarrow{a}\), \(\overrightarrow{b}\) und \(\overrightarrow{c}\) bilden in dieser Reihenfolge ein Rechtssystem. Rechtehandregel: Weist \(\overrightarrow{a}\) in Richtung des Daumens und \(\overrightarrow{b}\) in Richtung des Zeigefingers, dann weist \(\overrightarrow{c} = \overrightarrow{a} \times \overrightarrow{b}\) in Richtung des Mittelfingers.
Berechnung eines Vektorprodukts im \(\boldsymbol{\mathbb R^{3}}\) (vgl. Merkhilfe)
\[\overrightarrow{a} \times \overrightarrow{b} = \begin {pmatrix} a_1 \\ a_2 \\ a_3 \end {pmatrix} \times \begin {pmatrix} b_1 \\ b_2 \\ b_3 \end {pmatrix} = \begin {pmatrix} a_2 \cdot b_3 - a_3 \cdot b_2 \\ a_3 \cdot b_1 - a_1 \cdot b_3 \\ a_1 \cdot b_2 - a_2 \cdot b_1 \end {pmatrix}\]
\[\begin{align*}\overrightarrow{GE} \times \overrightarrow{GB} &= \begin {pmatrix} -6 \\ -6 \\ 0 \end {pmatrix} \times \begin {pmatrix} 0 \\ -6 \\ -6 \end {pmatrix} \\[0.8em] &= \begin {pmatrix} (-6) & \cdot & (-6) & - & 0 & \cdot & (-6) \\ 0 & \cdot & 0 & - & (-6) & \cdot & (-6) \\ (-6) & \cdot & (-6) & - & (-6) & \cdot & 0 \end {pmatrix} \\[0.8em] &= \begin {pmatrix} 36 \\ -36 \\ 36 \end {pmatrix} \\[0.8em] &= 36 \cdot \begin {pmatrix} 1 \\ -1 \\ 1 \end {pmatrix} \end{align*}\]
\[\Longrightarrow \quad \overrightarrow {n}_{L} = \begin {pmatrix} 1 \\ -1 \\ 1 \end {pmatrix}\]
Ebenengleichung in Normalenform in Vektordarstellung aufstellen:
Es sei \(G\,(6|6|6)\) Aufpunkt der Ebene \(L\).
Ebenengleichung in Normalenform (vgl. Merkhilfe)
Jede Ebene lässt sich durch eine Gleichung in Normalenform beschreiben. Ist \(A\) ein beliebiger Aufpunkt der Ebene \(E\) und \(\overrightarrow{n}_{E}\) ein Normalenvektor von \(E\), so erfüllt jeder Punkt \(X\) der Ebene \(E\) folgende Gleichungen:
Normalenform in Vektordarstellung
\[E \colon \overrightarrow{n}_{E} \circ (\overrightarrow{X} - \overrightarrow{A}) = 0\]

Normalenform in Koordinatendarstellung
\[E \colon n_{1}x_{1} + n_{2}x_{2} + n_{3}x_{3} + n_{0} = 0\]
mit \(n_{0} = -(\overrightarrow{n}_{E} \circ \overrightarrow{A}) = - \: n_{1}a_{1} - n_{2}a_{2} - n_{3}a_{3}\)
\(n_{1}\), \(n_{2}\) und \(n_{3}\): Koordinaten eines Normalenvektors \(\overrightarrow{n}_{E}\)
\[\begin {align*}L\, \colon & & \overrightarrow {n}_{L} \circ \left( \overrightarrow X - \overrightarrow {G} \right) &= 0 \\[0.8em] & & \begin {pmatrix} 1 \\ -1 \\ 1 \end {pmatrix} \circ \left[ \overrightarrow X - \begin {pmatrix} 6 \\ 6 \\ 6 \end {pmatrix} \right] &= 0 \end {align*}\]
Ebenengleichung in Normalenform in Koordinatendarstellung (Koordinatenform) bestimmen:
Skalarprodukt
Unter dem Skalarprodukt \(\overrightarrow{a} \circ \overrightarrow{b}\) zweier Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) versteht man das Produkt aus den Beträgen der beiden Vektoren und dem Kosinus des von den Vektoren eingeschlossenen Winkels \(\varphi\).
\[\overrightarrow{a} \circ \overrightarrow{b} = \vert \overrightarrow{a} \vert \cdot \vert \overrightarrow{b} \vert \cdot \cos{\varphi} \quad (0^{\circ} \leq \varphi \leq 180^{\circ})\]
Berechnung eines Skalarprodukts im \(\boldsymbol{\mathbb R^{3}}\) (vgl. Merkhilfe)
\[\overrightarrow{a} \circ \overrightarrow{b} = \begin{pmatrix} a_{1} \\ a_{2} \\ a_{3} \end{pmatrix} \circ \begin{pmatrix} b_{1} \\ b_{2} \\ b_{3} \end{pmatrix} = a_{1}b_{1} + a_{2}b_{2} + a_{3}b_{3}\]
\[ \begin {align*} \begin {pmatrix} 1 \\ -1 \\ 1 \end {pmatrix} \circ \left[ \overrightarrow X - \begin {pmatrix} 6 \\ 6 \\ 6 \end {pmatrix} \right] &= 0 \\[0.8em] 1 \cdot (x_1 - 6) + (-1) \cdot (x_2 - 6) + 1 \cdot (x_3 - 6) &= 0 \\[0.8em] x_1 - x_2 + x_3 - 6 &= 0 \end {align*} \]
\[L\, \colon \, x_1 - x_2 + x_3 = 6 \]
Es ist ebenso möglich, direkt mit der Normalenform in Koordinatendarstellung anzusetzen.
Ebenengleichung in Normalenform (vgl. Merkhilfe)
Jede Ebene lässt sich durch eine Gleichung in Normalenform beschreiben. Ist \(A\) ein beliebiger Aufpunkt der Ebene \(E\) und \(\overrightarrow{n}_{E}\) ein Normalenvektor von \(E\), so erfüllt jeder Punkt \(X\) der Ebene \(E\) folgende Gleichungen:
Normalenform in Vektordarstellung
\[E \colon \overrightarrow{n}_{E} \circ (\overrightarrow{X} - \overrightarrow{A}) = 0\]

Normalenform in Koordinatendarstellung
\[E \colon n_{1}x_{1} + n_{2}x_{2} + n_{3}x_{3} + n_{0} = 0\]
mit \(n_{0} = -(\overrightarrow{n}_{E} \circ \overrightarrow{A}) = - \: n_{1}a_{1} - n_{2}a_{2} - n_{3}a_{3}\)
\(n_{1}\), \(n_{2}\) und \(n_{3}\): Koordinaten eines Normalenvektors \(\overrightarrow{n}_{E}\)
\[{n}_{L} = \begin {pmatrix} 1 \\ -1 \\ 1 \end {pmatrix}\]
\(G\,(6|6|6) \in L\) (Aufpunkt)
\[\begin{align*}L\,\colon & & n_{1}x_{1} + n_{2}x_{2} + n_{3}x_{3} + n_{0} &= 0 & & (\text{mit}\; n_{0} = -n_{1}g_{1} - n_{2}g_{2} - n_{3}g_{3}) \\[0.8em] & & 1 \cdot x_{1} + (-1) \cdot x_{2} + 1 \cdot x_{3} + n_{0} &= 0 \\[0.8em] & & x_{1} - x_{2} +x_{3} + n_{0} &= 0 \end{align*}\]
\[\begin{align*} G \in L\,\colon\, 6 - 6 + 6 + n_{0} &= 0 \\[0.8em] 6 + n_{0} &= 0 & &| - 6 \\[0.8em] n_{0} &= -6 \end{align*}\]
\[L\, \colon \, x_1 - x_2 + x_3 = 6 \]
Zeichnung der Figur in der die Ebene \(L\) den Würfel schneidet
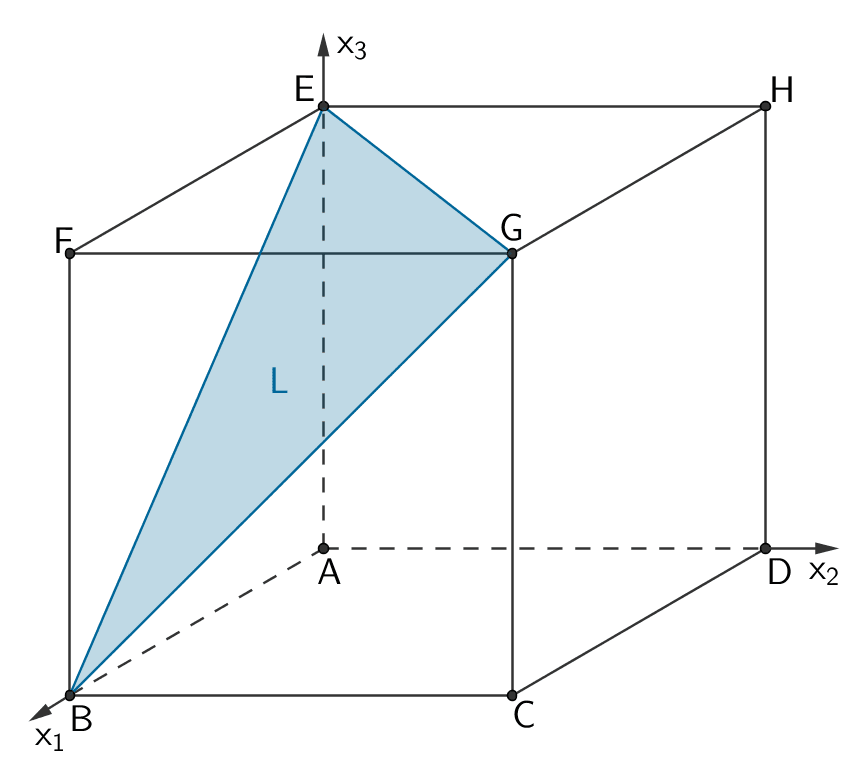
Figur (gleichseitiges Dreieck), in der die Ebne \(L\) den Würfel schneidet.


