Der Graph von \(f\), die \(x\)-Achse sowie die Geraden mit den Gleichungen \(x = 10\) und \(x = s\) mit \(s > 10\) schließen ein Flächenstück mit dem Inhalt \(A(s)\) ein. Bestimmen Sie \(A(s)\).
(Ergebnis: \(\displaystyle A(s) = 10 \cdot \ln{\frac{s^2 - 25}{75}}\))
(5 BE)
Lösung zu Teilaufgabe 1e
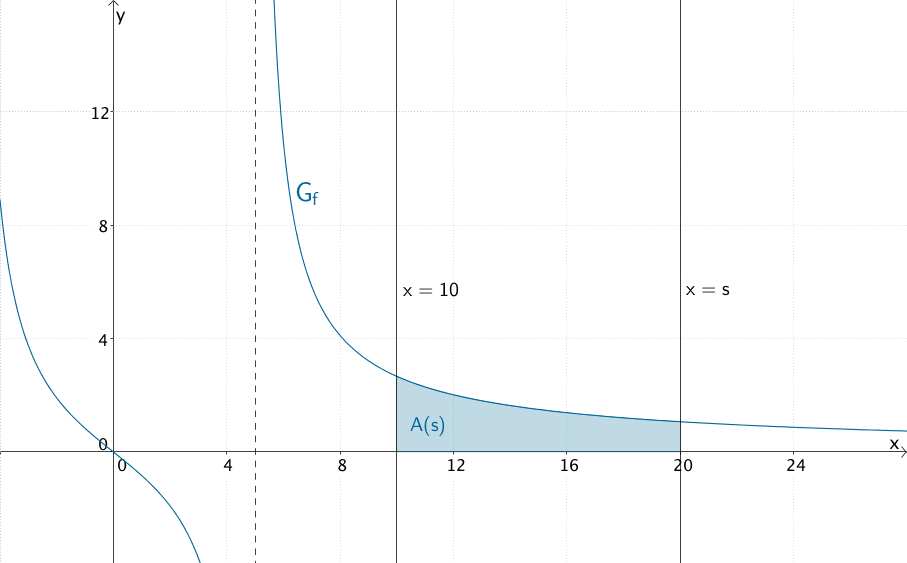
Flächenstück mit dem Inhalt \(A(s)\), welches \(G_{f}\), die \(x\)-Achse sowie die Geraden \(x = 10\) und \(x = s\,; \; s > 10\) einschließen.
Das bestimmte Integral \(\displaystyle \int_{10}^{s} f(x)\,dx\) errechnet den Flächeninhalt \(A(s)\).
\[A(s) = \int_{10}^{s} f(x)\,dx\]
Berechnung bestimmter Integrale
\[\int_{a}^{b} f(x)\,dx = [F(x)]_{a}^{b} = F(b) - F(a)\]
Dabei ist \(F\) eine beliebige Stammfunktion zu \(f\).
(vgl. Merkhilfe)
Eigenschaften des bestimmten Integrals - Integrationsregeln
Identische Integrationsgrenzen:
\[\int_{a}^{a} f(x)\,dx = 0\]
Faktorregel:
\(\displaystyle \int_{a}^{b} c \cdot f(x)\,dx = c \cdot \int_{a}^{b} f(x)\,dx\) mit \(c \in \mathbb R\)
Summenregel:
\[\int_{a}^{b} \left[f(x) \pm g(x) \right] dx = \int_{a}^{b}f(x)\,dx \pm \int_{a}^{b}g(x)\,dx\]
Vertauschungsregel:
\[\int_{a}^{b}f(x)\,dx = -\int_{b}^{a}f(x)\,dx\]
Zerlegung in Teilintervalle:
\(\displaystyle \int_{a}^{b}f(x)\,dx = \int_{a}^{c}f(x)\,dx + \int_{c}^{b}f(x)\,dx\) mit \(a \leq c \leq b\)
Stammfunktion \(F\) von \(f\):
Um eine Stammfunktion \(F\) von \(f\) bilden zu können, wird die Integrandenfunktion \(f\) so umgeformt, dass das unbestimmte Integral \(\displaystyle \int \frac{f'(x)}{f(x)}\,dx = \ln{\vert f(x) \vert} + C\) darauf angewendet werden kann.
\[f(x) = \frac{20x}{x^2 - 25} = 10 \cdot \frac{2x}{x^2 - 25}\]
Flächeninhalt \(A(s)\) bestimmen:
Wichtiges unbestimmtes Integral:
\[\int \frac{f'(x)}{f(x)}dx = \ln \vert f(x) \vert + C\]
(vgl. Merkhilfe)
\[\begin{align*} A(s) &= \int_{10}^{s} f(x)\,dx \\[0.8em] &= \int_{10}^{s} \left( \frac{20x}{x^2 - 25} \right) dx \\[0.8em] &= \int_{10}^{s} \left( 10 \cdot \frac{2x}{x^2 - 25} \right) dx \\[0.8em] &= 10 \cdot \int_{10}^{s} \left( \frac{2x}{x^2 - 25} \right) dx \\[0.8em] &= 10 \cdot \left[ \ln {\vert x^2 - 25 \vert} \right]_{10}^{s} \\[0.8em] &= 10 \cdot \left[ \ln{\vert s^2 - 25 \vert} - \left( \ln{\vert 10^2 - 25 \vert} \right) \right] \\[0.8em] &= 10 \cdot \left[ \ln{(s^2 - 25)} - \ln{75} \right] \\[0.8em] &= 10 \cdot \ln{\frac{s^2 - 25}{75}} \end{align*}\]


