Die Vektoren \(\overrightarrow{a} = \begin{pmatrix} 2 \\ 1 \\ 2 \end{pmatrix}\), \(\overrightarrow{b} = \begin{pmatrix} -1 \\ 2 \\ 0 \end{pmatrix}\) und \(\overrightarrow{c_t} = \begin{pmatrix} 4t \\ 2t \\ -5t \end{pmatrix}\) spannen für jeden Wert \(t\) mit \(t \in \mathbb R \,\backslash\,\{0\}\) einen Körper auf. Die Abbildung zeigt den Sachverhalt beispielhaft für einen Wert von \(t\).
Zeigen Sie, dass die aufgespannten Körper Quader sind.
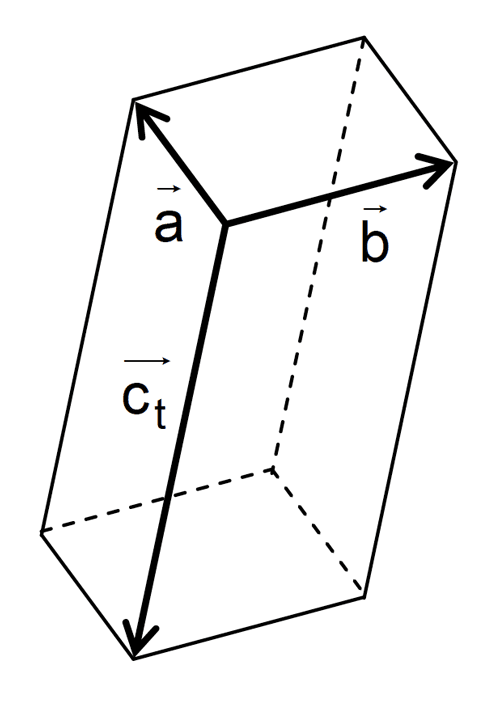
(2 BE)
Lösung zu Teilaufgabe 1a
\(\overrightarrow{a} = \begin{pmatrix} 2 \\ 1 \\ 2 \end{pmatrix}\), \(\overrightarrow{b} = \begin{pmatrix} -1 \\ 2 \\ 0 \end{pmatrix}\), \(\overrightarrow{c_t} = \begin{pmatrix} 4t \\ 2t \\ -5t \end{pmatrix}\)
Die aufgespannten Körper sind Quader, wenn die Vektoren \(\overrightarrow{a}\), \(\overrightarrow{b}\) und \(\overrightarrow{c_{t}}\) paarweise zueinander senkrecht sind.
\[\overrightarrow{a} \perp \overrightarrow{b}\,, \quad \overrightarrow{c_{t}} \perp \overrightarrow{a}\,, \quad \overrightarrow{c_{t}} \perp \overrightarrow{b}\]

Nachweis, dass \(\overrightarrow{a} \perp \overrightarrow{b}\), \(\overrightarrow{a} \perp \overrightarrow{c_{t}}\) und \(\overrightarrow{b} \perp \overrightarrow{c_{t}}\) gilt:
Anwendung des Skalarprodukts:
Orthogonale (zueinander senkrechte) Vektoren (vgl. Merkhilfe)
\[\overrightarrow{a} \perp \overrightarrow{b} \; \Leftrightarrow \; \overrightarrow{a} \circ \overrightarrow{b} \quad (\overrightarrow{a} \neq \overrightarrow{0},\; \overrightarrow{b} \neq \overrightarrow{0})\]
\[\overrightarrow{a} \circ \overrightarrow{b} = 0 \quad \Longleftrightarrow \quad \overrightarrow{a} \perp \overrightarrow{b}\]
\[\overrightarrow{a} \circ \overrightarrow{c_{t}} = 0 \quad \Longleftrightarrow \quad \overrightarrow{a} \perp \overrightarrow{c_{t}}\]
\[\overrightarrow{b} \circ \overrightarrow{c_{t}} = 0 \quad \Longleftrightarrow \quad \overrightarrow{b} \perp \overrightarrow{c_{t}}\]
Skalarprodukt
Unter dem Skalarprodukt \(\overrightarrow{a} \circ \overrightarrow{b}\) zweier Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) versteht man das Produkt aus den Beträgen der beiden Vektoren und dem Kosinus des von den Vektoren eingeschlossenen Winkels \(\varphi\).
\[\overrightarrow{a} \circ \overrightarrow{b} = \vert \overrightarrow{a} \vert \cdot \vert \overrightarrow{b} \vert \cdot \cos{\varphi} \quad (0^{\circ} \leq \varphi \leq 180^{\circ})\]
Berechnung eines Skalarprodukts im \(\boldsymbol{\mathbb R^{3}}\) (vgl. Merkhilfe)
\[\overrightarrow{a} \circ \overrightarrow{b} = \begin{pmatrix} a_{1} \\ a_{2} \\ a_{3} \end{pmatrix} \circ \begin{pmatrix} b_{1} \\ b_{2} \\ b_{3} \end{pmatrix} = a_{1}b_{1} + a_{2}b_{2} + a_{3}b_{3}\]
\[\begin{align*} \overrightarrow{a} \circ \overrightarrow{b} &= \begin{pmatrix} 2 \\ 1 \\ 2 \end{pmatrix} \circ \begin{pmatrix} -1 \\ 2 \\ 0 \end{pmatrix} \\[0.8em] &= 2 \cdot (-1) + 1 \cdot 2 + 2 \cdot 0 \\[0.8em] &= -2 + 2 = 0 \end{align*}\]
\[\Longrightarrow \quad \overrightarrow{a} \perp \overrightarrow{b}\]
\[\begin{align*} \overrightarrow{a} \circ \overrightarrow{c_{t}} &= \begin{pmatrix} 2 \\ 1 \\ 2 \end{pmatrix} \circ \begin{pmatrix} 4t \\ 2t \\ -5t \end{pmatrix} \\[0.8em] &= 2 \cdot 4t + 1 \cdot 2t + 2 \cdot (-5t) \\[0.8em] &= 8t + 2t - 10t = 0 \end{align*}\]
\[\Longrightarrow \quad \overrightarrow{a} \perp \overrightarrow{c_{t}}\]
\[\begin{align*} \overrightarrow{b} \circ \overrightarrow{c_{t}} &= \begin{pmatrix} -1 \\ 2 \\ 0 \end{pmatrix} \circ \begin{pmatrix} 4t \\ 2t \\ -5t \end{pmatrix} \\[0.8em] &= (-1) \cdot 4t + 2 \cdot 2t + 0 \cdot (-5t) \\[0.8em] &= -4t + 4t = 0 \end{align*}\]
\[\Longrightarrow \quad \overrightarrow{b} \perp \overrightarrow{c_{t}}\]
Die von den Vektoren \(\overrightarrow{a}\), \(\overrightarrow{b}\) und \(\overrightarrow{c_{t}}\) aufgespannten Körper sind für jeden Wert \(t\) mit \(t \in \mathbb R \,\backslash \, \{0\}\) Quader.
Alternative
Anstatt wiederholt zu zeigen, dass das Skalarprodukt der Vektoren \(\overrightarrow{a}\), \(\overrightarrow{b}\) und \(\overrightarrow{c_{t}}\) paarweise gleich Null ist, ist es ebenso möglich, das Vektorprodukt in den Lösungsweg mit einzubeziehen.
Die Orthogonalität der Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) sei an dieser Stelle bereits mithilfe des Skalarprodukts nachgewiesen.
Nachweis, dass \(\overrightarrow{c_{t}} \perp \overrightarrow{a}\) und \(\overrightarrow{c_{t}} \perp \overrightarrow{b}\) gilt:
Das Vektorprodukt \(\overrightarrow{a} \times \overrightarrow{b}\) beschreibt einen Vektor, der senkrecht zu den Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) ist.
Es ist zu zeigen, dass \(\overrightarrow{a} \times \overrightarrow{b} \in \overrightarrow{c_{t}}\) gilt, denn daraus folgt: \(\overrightarrow{c_{t}} \perp \overrightarrow{a}\) und \(\overrightarrow{c_{t}} \perp \overrightarrow{b}\).
Vektorprodukt (Kreuzprodukt)
Das Vektorprodukt \(\overrightarrow{a} \times \overrightarrow{b}\) zweier Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) erzeugt einen neuen Vektor \(\overrightarrow{c} = \overrightarrow{a} \times \overrightarrow{b}\) mit den Eigenschaften:
\(\overrightarrow{c}\) ist sowohl zu \(\overrightarrow{a}\) als auch zu \(\overrightarrow{b}\) senkrecht.
\[\overrightarrow{c} = \overrightarrow{a} \times \overrightarrow{b} \quad \Longrightarrow \quad \overrightarrow{c} \perp \overrightarrow{a}, \enspace \overrightarrow{c} \perp \overrightarrow{b}\]
Der Betrag des Vektorprodukts zweier Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) ist gleich dem Produkt aus den Beträgen der Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) und dem Sinus des von ihnen eingeschlossenen Winkels \(\varphi\).
\[\vert \overrightarrow{a} \times \overrightarrow{b} \vert = \vert \overrightarrow{a} \vert \cdot \vert \overrightarrow{b} \vert \cdot \sin{\varphi} \quad (0^{\circ} \leq \varphi \leq 180^{\circ})\]
Die Vektoren \(\overrightarrow{a}\), \(\overrightarrow{b}\) und \(\overrightarrow{c}\) bilden in dieser Reihenfolge ein Rechtssystem. Rechtehandregel: Weist \(\overrightarrow{a}\) in Richtung des Daumens und \(\overrightarrow{b}\) in Richtung des Zeigefingers, dann weist \(\overrightarrow{c} = \overrightarrow{a} \times \overrightarrow{b}\) in Richtung des Mittelfingers.
Berechnung eines Vektorprodukts im \(\boldsymbol{\mathbb R^{3}}\) (vgl. Merkhilfe)
\[\overrightarrow{a} \times \overrightarrow{b} = \begin {pmatrix} a_1 \\ a_2 \\ a_3 \end {pmatrix} \times \begin {pmatrix} b_1 \\ b_2 \\ b_3 \end {pmatrix} = \begin {pmatrix} a_2 \cdot b_3 - a_3 \cdot b_2 \\ a_3 \cdot b_1 - a_1 \cdot b_3 \\ a_1 \cdot b_2 - a_2 \cdot b_1 \end {pmatrix}\]
\[\begin{align*} \overrightarrow{a} \times \overrightarrow{b} &= \begin{pmatrix} 2 \\ 1 \\ 2 \end{pmatrix} \times \begin{pmatrix} -1 \\ 2 \\ 0 \end{pmatrix} \\[0.8em] &= \begin{pmatrix} 1 & \cdot & 0 & - & 2 & \cdot & 2 \\ 2 & \cdot & (-1) & - & 2 & \cdot & 0 \\ 2 & \cdot & 2 & - & 1 & \cdot & (-1) \end{pmatrix} \\[0.8em] &= \begin{pmatrix} -4 \\ -2 \\ 5 \end{pmatrix} \\[0.8em] &= (-1) \cdot \begin{pmatrix} 4 \\ 2 \\ -5 \end{pmatrix} \end{align*}\]
\[\overrightarrow{c_{t}} = \begin{pmatrix} 4t \\ 2t \\ -5t \end{pmatrix} = t \cdot \begin{pmatrix} 4 \\ 2 \\ -5 \end{pmatrix}\,; \quad t \in \mathbb R \, \backslash \, \{0\}\]
\[\Longrightarrow \quad \overrightarrow{a} \times \overrightarrow{b} \in \overrightarrow{c_{t}}\]
\[\Longrightarrow \quad \overrightarrow{c_{t}} \perp \overrightarrow{a}\,, \enspace \overrightarrow{c_{t}} \perp \overrightarrow{b}\]
Die von den Vektoren \(\overrightarrow{a}\), \(\overrightarrow{b}\) und \(\overrightarrow{c_{t}}\) aufgespannten Körper sind für jeden Wert \(t\) mit \(t \in \mathbb R \,\backslash \, \{0\}\) Quader.


