Gegeben ist die Gerade \(g \colon \overrightarrow{X} = \begin{pmatrix} 1 \\ 7 \\ 2 \end{pmatrix} + \lambda \cdot \begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix}\), \(\lambda \in \mathbb R\), sowie eine weitere Gerade \(h\), welche parallel zu \(g\) ist und durch den Punkt \(A(2|0|0)\) verläuft. Der Punkt \(B\) liegt auf \(g\) so, dass die Geraden \(AB\) und \(h\) senkrecht zueinander sind.
Bestimmen Sie die Koordinaten von \(B\).
(zur Kontrolle: \(B(-2|3|2)\))
(4 BE)
Lösung zu Teilaufgabe a
\[g \colon \overrightarrow{X} = \begin{pmatrix} 1 \\ 7 \\ 2 \end{pmatrix} + \lambda \cdot \begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix}, \;\lambda \in \mathbb R\]
\(h \parallel g\), \(A(2|0|0) \in h\), \(B \in g\), \(AB \perp h\)
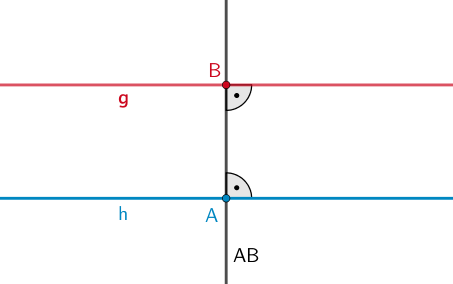
Planskizze (optional): Der Punkt \(\textcolor{#cc071e}{B \in g}\) ist der Lotfußpunkt des Lotes des Punktes \(\textcolor{#0087c1}{A}\) auf die Gerade \(\textcolor{#cc071e}{g}\).
Drei mögliche Lösungsansätze für die Bestimmung der Koordinaten von \(B\):
1. Möglichkeit: Skalarprodukt orthogonaler Vektoren anwenden
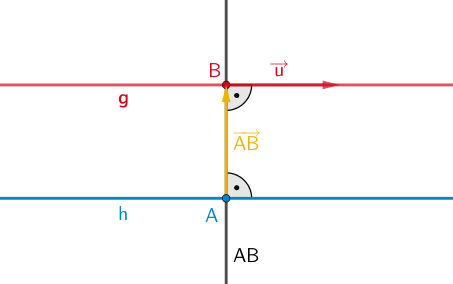
Planskizze (optional): Der Verbindungsvektor \(\textcolor{#e9b509}{\overrightarrow{AB}}\) und der Richtungsvektor \(\textcolor{#cc071e}{\overrightarrow{u} = \begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix}}\) der Gerade \(\textcolor{#cc071e}{g}\) sind zueinander senkrecht (orthogonal). Folglich muss gelten:
Anwendung des Skalarprodukts:
Orthogonale (zueinander senkrechte) Vektoren (vgl. Merkhilfe)
\[\overrightarrow{a} \perp \overrightarrow{b} \; \Leftrightarrow \; \overrightarrow{a} \circ \overrightarrow{b} \quad (\overrightarrow{a} \neq \overrightarrow{0},\; \overrightarrow{b} \neq \overrightarrow{0})\]
\[\textcolor{#e9b509}{\overrightarrow{AB}} \perp \textcolor{#cc071e}{\overrightarrow{u}} \enspace \Leftrightarrow \enspace \textcolor{#e9b509}{\overrightarrow{AB}} \circ \textcolor{#cc071e}{\overrightarrow{u}} = 0\]
Zunächst wird der Verbindungsvektor \(\textcolor{#e9b509}{\overrightarrow{AB}}\) in Abhängigkeit des Parameters \(\textcolor{#cc071e}{\lambda}\) der Gleichung der Gerade \(\textcolor{#cc071e}{g}\) formuliert.
\[\textcolor{#cc071e}{B \in g \colon \overrightarrow{B}} = \begin{pmatrix} 1 \\ 7 \\ 2 \end{pmatrix} + \lambda \cdot \begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix} = \textcolor{#cc071e}{\begin{pmatrix} 1 + 3\lambda \\ 7 + 4\lambda \\ 2 \end{pmatrix}}, \;\lambda \in \mathbb R\]
\[\textcolor{#0087c1}{\overrightarrow{A} = \begin{pmatrix} 2 \\ 0 \\ 0 \end{pmatrix}}\]
\[\textcolor{#e9b509}{\overrightarrow{AB}} = \textcolor{#cc071e}{\overrightarrow{B}} - \textcolor{#0087c1}{\overrightarrow{A}} = \textcolor{#cc071e}{\begin{pmatrix} 1 + 3\lambda \\ 7 + 4\lambda \\ 2 \end{pmatrix}} - \textcolor{#0087c1}{\begin{pmatrix} 2 \\ 0 \\ 0 \end{pmatrix}} = \textcolor{#e9b509}{\begin{pmatrix} -1 + 3\lambda \\ 7 + 4\lambda \\ 2\end{pmatrix}}\]
Wendet man nun das Skalarprodukt der orthogonalen Vektoren \(\textcolor{#e9b509}{\overrightarrow{AB}}\) und \(\textcolor{#cc071e}{\overrightarrow{u} = \begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix}}\) an, so liefert die Lösung der Gleichung denjenigen Wert des Parameters \(\textcolor{#cc071e}{\lambda}\), der eingesetzt in die Gleichung der Gerade \(\textcolor{#cc071e}{g}\) den Ortsvektor \(\textcolor{#cc071e}{\overrightarrow{B}}\) ergibt.
Skalarprodukt
Unter dem Skalarprodukt \(\overrightarrow{a} \circ \overrightarrow{b}\) zweier Vektoren \(\overrightarrow{a}\) und \(\overrightarrow{b}\) versteht man das Produkt aus den Beträgen der beiden Vektoren und dem Kosinus des von den Vektoren eingeschlossenen Winkels \(\varphi\).
\[\overrightarrow{a} \circ \overrightarrow{b} = \vert \overrightarrow{a} \vert \cdot \vert \overrightarrow{b} \vert \cdot \cos{\varphi} \quad (0^{\circ} \leq \varphi \leq 180^{\circ})\]
Berechnung eines Skalarprodukts im \(\boldsymbol{\mathbb R^{3}}\) (vgl. Merkhilfe)
\[\overrightarrow{a} \circ \overrightarrow{b} = \begin{pmatrix} a_{1} \\ a_{2} \\ a_{3} \end{pmatrix} \circ \begin{pmatrix} b_{1} \\ b_{2} \\ b_{3} \end{pmatrix} = a_{1}b_{1} + a_{2}b_{2} + a_{3}b_{3}\]
\[\begin{align*}\textcolor{#e9b509}{\overrightarrow{AB}} \circ \textcolor{#cc071e}{\overrightarrow{u}} &= 0 \\[0.8em] \textcolor{#e9b509}{\begin{pmatrix} -1 + 3\lambda \\ 7 + 4\lambda \\ 2\end{pmatrix}} \circ \textcolor{#cc071e}{\begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix}} &= 0 \\[0.8em] \textcolor{#e9b509}{(-1 + 3\lambda)} \cdot \textcolor{#cc071e}{3} + \textcolor{#e9b509}{(7 + 4\lambda)} \cdot \textcolor{#cc071e}{4} + \textcolor{#e9b509}{2} \cdot \textcolor{#cc071e}{0} &= 0 \\[0.8em] -3 + 9\lambda + 28 + 16\lambda &= 0 \\[0.8em] 25\lambda + 25 &= 0 &&| - 25 \\[0.8em] 25\lambda &= -25 &&| : 25 \\[0.8em] \lambda &= -1 \end{align*} \\[0.8em] \]
\[\textcolor{#cc071e}{B \in g \colon \overrightarrow{B}} = \begin{pmatrix} 1 + 3 \cdot (-1) \\ 7 + 4 \cdot (-1) \\ 2 \end{pmatrix} = \textcolor{#cc071e}{\begin{pmatrix} -2 \\ 3 \\ 2 \end{pmatrix}} \enspace \Rightarrow \enspace \textcolor{#cc071e}{B(-2|3|2)}\]
2. Möglichkeit: Hilfsebene aufstellen
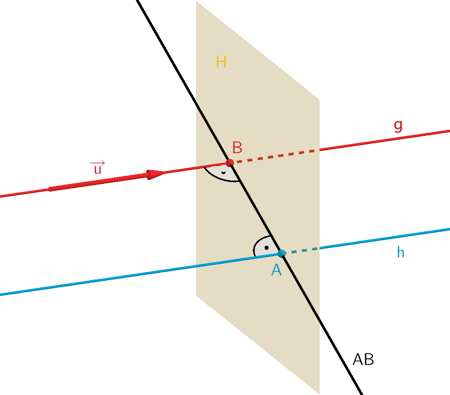
Planskizze (optional): Die Hilfsebene \(\textcolor{#e9b509}{H}\) mit den Eigenschaften \(\textcolor{#0087c1}{A} \in \textcolor{#0087c1}{h}\) und \(\textcolor{#cc071e}{g} \perp \textcolor{#e9b509}{H}\) schneidet die Gerade \(\textcolor{#cc071e}{g}\) im Punkt \(\textcolor{#cc071e}{B}\) (Lotfußpunkt des Lotes von \(\textcolor{#0087c1}{A}\) auf \(\textcolor{#cc071e}{g}\)).
Der Richtungsvektor \(\textcolor{#cc071e}{\overrightarrow{u} = \begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix}}\) ist ein Normalenvektor der Hilfsebene \(\textcolor{#e9b509}{H}\). Der Punkt \(\textcolor{#0087c1}{A(2|0|0)}\) dient als Aufpunkt für eine Gleichung von \(\textcolor{#e9b509}{H}\) in Normalenform.
Gleichung der Hilfsebene \(\textcolor{#e9b509}{H}\) in Normalenform in Koordinatendarstellung (Koordinatenform) aufstellen:
(Ansatz mit Vektordarstellung oder Koordinatendarstellung möglich)
Ebenengleichung in Normalenform (vgl. Merkhilfe)
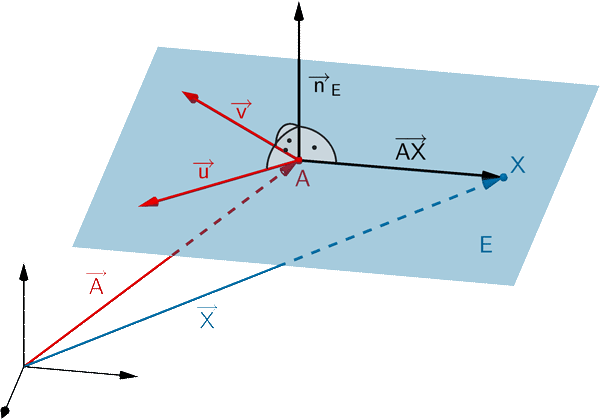
Jede Ebene lässt sich durch eine Gleichung in Normalenform beschreiben. Ist \(A\) ein beliebiger Aufpunkt der Ebene \(E\) und \(\overrightarrow{n}_{E}\) ein Normalenvektor von \(E\), so erfüllt jeder Punkt \(X\) der Ebene \(E\) folgende Gleichungen:
Normalenform in Vektordarstellung
\[E \colon \overrightarrow{n}_{E} \circ (\overrightarrow{X} - \overrightarrow{A}) = 0\]
Normalenform in Koordinatendarstellung
\[E \colon n_{1}x_{1} + n_{2}x_{2} + n_{3}x_{3} + n_{0} = 0\]
mit \(n_{0} = -(\overrightarrow{n}_{E} \circ \overrightarrow{A}) = - \: n_{1}a_{1} - n_{2}a_{2} - n_{3}a_{3}\)
\(n_{1}\), \(n_{2}\) und \(n_{3}\): Koordinaten eines Normalenvektors \(\overrightarrow{n}_{E}\)
\[\begin{align*} \textcolor{#e9b509}{H} \colon \textcolor{#cc071e}{\overrightarrow{u}} \circ \Big( \overrightarrow{X} - \textcolor{#0087c1}{\overrightarrow{A}} \Big) &= 0 \\[0.8em] \textcolor{#cc071e}{\begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix}} \circ \left[ \overrightarrow{X} - \textcolor{#0087c1}{\begin{pmatrix} 2 \\ 0 \\ 0 \end{pmatrix}} \right] &= 0 \\[0.8em] \textcolor{#cc071e}{3} \cdot (x_{1} - \textcolor{#0087c1}{2}) + \textcolor{#cc071e}{4} \cdot (x_{2} - \textcolor{#0087c1}{0}) + \textcolor{#cc071e}{0} \cdot (x_{3} - \textcolor{#0087c1}{0}) &= 0 \\[0.8em] 3x_{1} - 6 + 4x_{2} &= 0 \\[0.8em] \textcolor{#e9b509}{H} \colon 3x_{1} + 4x_{2} - 6 &= 0\end{align*}\]
oder
\[\begin{align*} \textcolor{#e9b509}{H} \colon \textcolor{#cc071e}{n_{1}}x_{1} + \textcolor{#cc071e}{n_{2}}x_{2} + \textcolor{#cc071e}{n_{3}}x_{3} + n_{0} &= 0 \\[0.8em] \textcolor{#cc071e}{3} \cdot x_{1} + \textcolor{#cc071e}{4} \cdot x_{2} + \textcolor{#cc071e}{0} \cdot x_{3} + n_{0} &= 0 \\[0.8em] \textcolor{#cc071e}{3}x_{1} + \textcolor{#cc071e}{4}x_{2} + n_{0} &= 0\end{align*}\]
\[\begin{align*}\textcolor{#0087c1}{A(2|0|0)} \in \textcolor{#e9b509}{H} \colon \textcolor{#cc071e}{3} \cdot \textcolor{#0087c1}{2} + \textcolor{#cc071e}{4} \cdot \textcolor{#0087c1}{0} + n_{0} &= 0 \\[0.8em] 6 + n_{0} &= 0 &&| - 6 \\[0.8em] n_{0} &= -6\end{align*}\]
\[\Rightarrow \enspace \textcolor{#e9b509}{H} \colon 3x_{1} + 4x_{2} - 6 = 0\]
Schnittpunkt \(\textcolor{#cc071e}{B}\) der Gerade \(\textcolor{#cc071e}{g}\) und der Hilfsebene \(\textcolor{#e9b509}{H}\) bestimmen:
Hierfür werden Die Koordinaten des Ortsvektors \(\overrightarrow{X}\) der Gleichung der Gerade \(\textcolor{#cc071e}{g}\) in die Gleichung der Hilfsebene \(\textcolor{#e9b509}{H}\) eingesetzt und diese nach dem Parameter \(\lambda\) aufgelöst.
\[\textcolor{#cc071e}{g \colon \overrightarrow{X}} = \begin{pmatrix} 1 \\ 7 \\ 2 \end{pmatrix} + \lambda \cdot \begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix} = \textcolor{#cc071e}{\begin{pmatrix} 1 + 3\lambda \\ 7 + 4\lambda \\ 2 \end{pmatrix}}, \;\lambda \in \mathbb R\]
\[\textcolor{#e9b509}{H} \colon 3x_{1} + 4x_{2} - 6 = 0\]
\[\begin{align*} \textcolor{#cc071e}{g} \cap \textcolor{#e9b509}{H} \colon 3 \cdot \textcolor{#cc071e}{(1 + 3\lambda)} + 4 \cdot \textcolor{#cc071e}{(7 + 4\lambda)} - 6 &= 0 \\[0.8em] 3 + 9\lambda + 28 + 16\lambda - 6 &= 0 \\[0.8em] 25\lambda + 25 &= 0 &&| - 25 \\[0.8em] 25\lambda &= -25 &&| : 25 \\[0.8em] \lambda &= -1 \end{align*}\]
Durch Einsetzen des Parameterwerts \(\lambda = -1\) in die Gleichung der Gerade \(\textcolor{#cc071e}{g}\) erhält man den Ortsvektor \(\textcolor{#cc071e}{\overrightarrow{B}}\).
\[\textcolor{#cc071e}{B \in g \colon \overrightarrow{B}} = \begin{pmatrix} 1 + 3 \cdot (-1) \\ 7 + 4 \cdot (-1) \\ 2 \end{pmatrix} = \textcolor{#cc071e}{\begin{pmatrix} -2 \\ 3 \\ 2 \end{pmatrix}} \enspace \Rightarrow \enspace \textcolor{#cc071e}{B(-2|3|2)}\]
3. Möglichkeit: Differentialrechnung anwenden
![Länge der Strecke [AX] zwischen Punkt A ∈ h und einem beliebigen Punkt X ∈ g](/images/stories/B2021_PT_A_G1/B2021_PT_A_G1_a4.png)
Planskizze (optional): Die Länge der Strecke \(\textcolor{#e9b509}{[AX]}\) zwischen dem Punkt \(\textcolor{#0087c1}{A \in h}\) und einem beliebigen Punkt \(\textcolor{#cc071e}{X \in g}\) lässt sich in Abhängigkeit des Parameters \(\textcolor{#cc071e}{\lambda}\) der Geradengleichung von \(\textcolor{#cc071e}{g}\) formulieren.
Die notwendige Bedingung für die kürzeste Strecke\(\textcolor{#e9b509}{[AX]}\) (Lot von \(\textcolor{#0087c1}{A}\) auf \(\textcolor{#cc071e}{g}\)) lautet \(\textcolor{#e9b509}{\overline{AX}'(\lambda) = 0}\). Die Lösung der Gleichung liefert denjenigen Wert des Parameters \(\textcolor{#cc071e}{\lambda}\), der eingesetzt in die Gleichung von \(\textcolor{#cc071e}{g}\) den Ortsvektor \(\textcolor{#cc071e}{\overrightarrow{B}}\) ergibt.
Verbindungsvektor \(\textcolor{#e9b509}{\overrightarrow{AX}}\) formulieren:
\[\textcolor{#cc071e}{g \colon \overrightarrow{X}} = \begin{pmatrix} 1 \\ 7 \\ 2 \end{pmatrix} + \lambda \cdot \begin{pmatrix} 3 \\ 4 \\ 0 \end{pmatrix} = \textcolor{#cc071e}{\begin{pmatrix} 1 + 3\lambda \\ 7 + 4\lambda \\ 2 \end{pmatrix}}, \;\lambda \in \mathbb R\]
\[\textcolor{#0087c1}{\overrightarrow{A} = \begin{pmatrix} 2 \\ 0 \\ 0 \end{pmatrix}}\]
\[\textcolor{#e9b509}{\overrightarrow{AX}} = \textcolor{#cc071e}{\overrightarrow{X}} - \textcolor{#0087c1}{\overrightarrow{A}} = \textcolor{#cc071e}{\begin{pmatrix} 1 + 3\lambda \\ 7 + 4\lambda \\ 2 \end{pmatrix}} - \textcolor{#0087c1}{\begin{pmatrix} 2 \\ 0 \\ 0 \end{pmatrix}} = \textcolor{#e9b509}{\begin{pmatrix} -1 + 3\lambda \\ 7 + 4\lambda \\ 2 \end{pmatrix}}\]
Länge der Strecke \(\textcolor{#e9b509}{[AX]}\) beschreiben:
Betrag eines Vektors
\[ \vert \overrightarrow{a} \vert = \sqrt{\overrightarrow{a} \circ \overrightarrow{a}} = \sqrt{{a_1}^2 + {a_2}^2 + {a_3}^2}\]
(vgl. Merkhilfe)
\[\begin{align*} \textcolor{#e9b509}{\overline{AX}(\lambda)} &= \vert \textcolor{#e9b509}{\overrightarrow{AX}} \vert = \left| \textcolor{#e9b509}{\begin{pmatrix} -1 + 3\lambda \\ 7 + 4\lambda \\ 2 \end{pmatrix}} \right| \\[0.8em] &= \sqrt{(\textcolor{#e9b509}{-1 + 3\lambda})^{2} + (\textcolor{#e9b509}{7 + 4\lambda})^{2} + \textcolor{#e9b509}{2}^{2}} &&| \; \text{1. Binom. Formel} \\[0.8em] &= \sqrt{1 - 6\lambda + 9\lambda^{2} + 49 + 56\lambda + 16\lambda^{2} + 4} \\[0.8em] &= \sqrt{25\lambda^{2} + 50\lambda + 54} \end{align*}\]
Erste Ableitung \(\textcolor{#e9b509}{\overline{AX}'(\lambda)}\) bilden:
Hierfür wird die Ableitung einer Wurzelfunktion, die Kettenregel sowie die Ableitung einer Potenzfunktion benötigt. Als Alternative formuliert man \(\textcolor{#e9b509}{\overline{AX}(\lambda)}\) mithilfe des Potenzgesetzes \(a^{\frac{m}{n}} = \sqrt[n]{a^{m}}\) (vgl. Merkhilfe) in der Potenzschreibweise und wendet die Ableitung einer Potenzfunktion sowie die Kettenregel an.
\[\textcolor{#e9b509}{\overline{AX}(\lambda)} = \sqrt{25\lambda^{2} + 50\lambda + 54} = (25\lambda^{2} + 50\lambda + 54)^{\frac{1}{2}}\]

Ableitungen der Grundfunktionen
\[c' = 0 \enspace (c \in \mathbb R)\]
\[\left( x^r \right)' = r \cdot x^{r - 1} \enspace (r \in \mathbb R)\]
\[\left( \sqrt{x} \right)' = \frac{1}{2\sqrt{x}}\]
\[\left( \sin{x} \right)' = \cos{x}\]
\[\left( \cos{x} \right)' = -\sin{x}\]
\[\left( \ln{x} \right)' = \frac{1}{x}\]
\[\left( \log_{a}{x}\right)' = \frac{1}{x \cdot \ln{a}}\]
\[\left( e^x \right)' = e^x\]
\[\left(a^x \right)' = a^x \cdot \ln{a}\]
Faktorregel
\[\begin{align*}f(x) &= a \cdot \textcolor{#0087c1}{u(x)} \\[0.8em] f'(x) &= a \cdot \textcolor{#0087c1}{u'(x)}\end{align*}\]
Summenregel
\[\begin{align*}f(x) &= \textcolor{#0087c1}{u(x)} + \textcolor{#cc071e}{v(x)} \\[0.8em] f'(x) &= \textcolor{#0087c1}{u'(x)} + \textcolor{#cc071e}{v'(x)}\end{align*}\]
Produktregel
\[\begin{align*}f(x) &= \textcolor{#0087c1}{u(x)} \cdot \textcolor{#cc071e}{v(x)} \\[0.8em] f'(x) &= \textcolor{#0087c1}{u'(x)} \cdot \textcolor{#cc071e}{v(x)} + \textcolor{#0087c1}{u(x)} \cdot \textcolor{#cc071e}{v'(x)}\end{align*}\]
Quotientenregel
\[\begin{align*}f(x) &= \dfrac{\textcolor{#0087c1}{u(x)}}{\textcolor{#cc071e}{v(x)}} \\[0.8em] f'(x) &= \dfrac{\textcolor{#0087c1}{u'(x)} \cdot \textcolor{#cc071e}{v(x)} - \textcolor{#0087c1}{u(x)} \cdot \textcolor{#cc071e}{v'(x)}}{[\textcolor{#cc071e}{v(x)}]^{2}}\end{align*}\]
Kettenregel
\[\begin{align*}f(x) &= \textcolor{#0087c1}{u(}\textcolor{#cc071e}{v(x)}\textcolor{#0087c1}{)} \\[0.8em] f'(x) &= \textcolor{#0087c1}{u'(}\textcolor{#cc071e}{v(x)}\textcolor{#0087c1}{)} \cdot \textcolor{#cc071e}{v'(x)}\end{align*}\]
\[\begin{align*}\textcolor{#e9b509}{\overline{AX}'(\lambda)} &= \frac{1}{2\sqrt{25\lambda^{2} + 50\lambda + 54}} \cdot (50\lambda + 50) \\[0.8em] &= \frac{50\lambda + 50}{2\sqrt{25\lambda^{2} + 50\lambda + 54}}\end{align*}\]
oder
\[\begin{align*}\textcolor{#e9b509}{\overline{AX}'(\lambda)} &= \frac{1}{2}(25\lambda^{2} + 50\lambda + 54)^{-\frac{1}{2}} \cdot (50\lambda + 50) &&| \; a^{-n} = \frac{1}{a^{n}}; \; a^{\frac{m}{n}} = \sqrt[n]{a^{m}} \\[0.8em] &= \frac{50\lambda + 50}{2\sqrt{25\lambda^{2} + 50\lambda +54}}\end{align*}\]
Notwendige Bedingung \(\textcolor{#e9b509}{\overline{AX}'(\lambda) = 0}\) für die kürzeste Strecke \(\textcolor{#e9b509}{[AX]}\) formulieren:
\[\begin{align*} \textcolor{#e9b509}{\overline{AX}'(\lambda)} &\textcolor{#e9b509}{=} \textcolor{#e9b509}{0} \\[0.8em] \frac{50\lambda + 50}{2\sqrt{25\lambda^{2} + 50\lambda + 54}} &= 0 \\[0.8em] \Rightarrow \enspace 50\lambda + 50 &= 0 &&| - 50 \\[0.8em] 50\lambda &= -50 &&| : 50 \\[0.8em] \lambda &= -1\end{align*}\]
Durch Einsetzen des Parameterwerts \(\lambda = -1\) in die Gleichung der Gerade \(\textcolor{#cc071e}{g}\) erhält man den Ortsvektor \(\textcolor{#cc071e}{\overrightarrow{B}}\).
\[\textcolor{#cc071e}{B \in g \colon \overrightarrow{B}} = \begin{pmatrix} 1 + 3 \cdot (-1) \\ 7 + 4 \cdot (-1) \\ 2 \end{pmatrix} = \textcolor{#cc071e}{\begin{pmatrix} -2 \\ 3 \\ 2 \end{pmatrix}} \enspace \Rightarrow \enspace \textcolor{#cc071e}{B(-2|3|2)}\]


